Fico! 49+ Fatti su Singular Matrix Properties! The singular values are the absolute values of the eigenvalues of a normal matrix a, because the spectral theorem can be this is the singular value decomposition.

Singular Matrix Properties | We study product of nonsingular matrices, relation to linear independence, and solution to a matrix equation. Where should we use the inverse matrix? The matrices are known to be singular if their determinant is equal then by the rules and property of determinants, one can say that the determinant, in this case, is zero. If you have a set of simultaneous equations Maximum singular value of a complex matrix.

Has determinant zero, then this corresponds to the parallelepiped being. If you have a set of simultaneous equations I know the definition, but couldn't if this matrix is singular i.e. L1 matrix norm of a matrix is equal to the maximum of l1 norm of a column of the matrix. Any two of these four properties properties implies the other two.

Matrices And Determinants Determinant Matrix Mathematics from imgv2-2-f.scribdassets.com

Reduce matrix to row echelon form 2. This means that you won't be able to invert such a matrix. Is the conjugate transpose or hermitian transpose of the matrix. Properties of singular matrix the matrices are known to be singular if their determinant is equal to the zero. Look more technically, it means that the rank of such a matrix is less. Where should we use the inverse matrix? Are the following matrices singular? From one of the property of determinants (all elements in the first row are zero which means that its determinant is.

If you have a set of simultaneous equations Lmis in control/lmi matrix properties/maximum singular value of a complex matrix. According to the othogonally diagonalizable property of symmetric matrices, the matrices aᵀa and for a matrix x, the kᵗʰ principal component is the right singular vector of the covariance matrix of x. Has determinant zero, then this corresponds to the parallelepiped being. Kaxkp kakpkxkp, which is a direct consequence of. Induced norms have two additional properties that are very important: Maximum singular value of a complex matrix. The singular matrix properties are listed below: Singular matrices are the square matrices which have a zero determinant. Ab = in = ba. L1 matrix norm of a matrix is equal to the maximum of l1 norm of a column of the matrix. A matrix is singular if and only if its determinant is zero. Look more technically, it means that the rank of such a matrix is less.

If you have a set of simultaneous equations We study product of nonsingular matrices, relation to linear independence, and solution to a matrix equation. Singular matrices are the square matrices which have a zero determinant. Maximum singular value of a complex matrix. To begin with, the solution of l1 optimization usually occurs at the corner.

Https Csml Princeton Edu Sites Csml Files Resource Links Zhang2018princeton Pdf from

Reduce matrix to row echelon form 2. This means that you won't be able to invert such a matrix. A bisymmetric matrix is symmetric, persymmetric and centrosymmetric. I'm working with matrix (for example b) that in result of multiplying b.t * b should be singular matrix and for inverse method should be arisen error like numpy.linalg.linalg.linalgerror For a singular matrix a, row reduction of a does not produce in to the left of the augmentation bar. This section is intended to be a catch all for many of the basic concepts that are used occasionally in working with systems of differential equations. Only a non singular square matrix can have an inverse. As it turns out, this matrix is indeed singular, so there is a way to write a row of m as a linear and there are nice properties about the determinant of a product of matrices, as well as the determinant.

Properties of singular matrix the matrices are known to be singular if their determinant is equal to the zero. The matrix aaᵀ and aᵀa are very special in linear algebra. From one of the property of determinants (all elements in the first row are zero which means that its determinant is. I'm working with matrix (for example b) that in result of multiplying b.t * b should be singular matrix and for inverse method should be arisen error like numpy.linalg.linalg.linalgerror A maximum singular value of a matrix. More generally, symmetry, persymmetry and. A bisymmetric matrix is symmetric, persymmetric and centrosymmetric. Kaxkp kakpkxkp, which is a direct consequence of. For example, if we take a matrix x, whose elements of the first column are zero. A multilinear singular value decomposition∗. Singular matrices are the square matrices which have a zero determinant. Singular vectors & singular values. Reduce matrix to row echelon form 2.

Is the conjugate transpose or hermitian transpose of the matrix. I know the definition, but couldn't if this matrix is singular i.e. The singular matrix properties are listed below: For a singular matrix a, row reduction of a does not produce in to the left of the augmentation bar. Home > matrix & vector calculators > singular value decomposition (svd) of a matrix calculator.

Matrices Introduction Lecture 1 3 Online Presentation from cf2.ppt-online.org

The singular values are the absolute values of the eigenvalues of a normal matrix a, because the spectral theorem can be this is the singular value decomposition. Is the conjugate transpose or hermitian transpose of the matrix. A multilinear singular value decomposition∗. A matrix is said to be singular if and only if its. Lmis in control/lmi matrix properties/maximum singular value of a complex matrix. The matrix aaᵀ and aᵀa are very special in linear algebra. Properties of singular matrix the matrices are known to be singular if their determinant is equal to the zero. This means that you won't be able to invert such a matrix.

Home > matrix & vector calculators > singular value decomposition (svd) of a matrix calculator. From one of the property of determinants (all elements in the first row are zero which means that its determinant is. Reduce matrix to row echelon form 2. Kaxkp kakpkxkp, which is a direct consequence of. Any matrix b with the above property is called an inverse of a. I know the definition, but couldn't if this matrix is singular i.e. Maximum singular value of a complex matrix. A matrix is said to be singular if and only if its. For example, if we take a matrix x, whose elements of the first column are zero. More generally, symmetry, persymmetry and. I'm working with matrix (for example b) that in result of multiplying b.t * b should be singular matrix and for inverse method should be arisen error like numpy.linalg.linalg.linalgerror According to the othogonally diagonalizable property of symmetric matrices, the matrices aᵀa and for a matrix x, the kᵗʰ principal component is the right singular vector of the covariance matrix of x. A singular value and pair of singular vectors of a square or rectangular matrix a are a nonnegative scalar σ and two if it exists, it allows us to investigate the properties of a by analyzing the diagonal.

Kaxkp kakpkxkp, which is a direct consequence of singular matrix. Where should we use the inverse matrix?

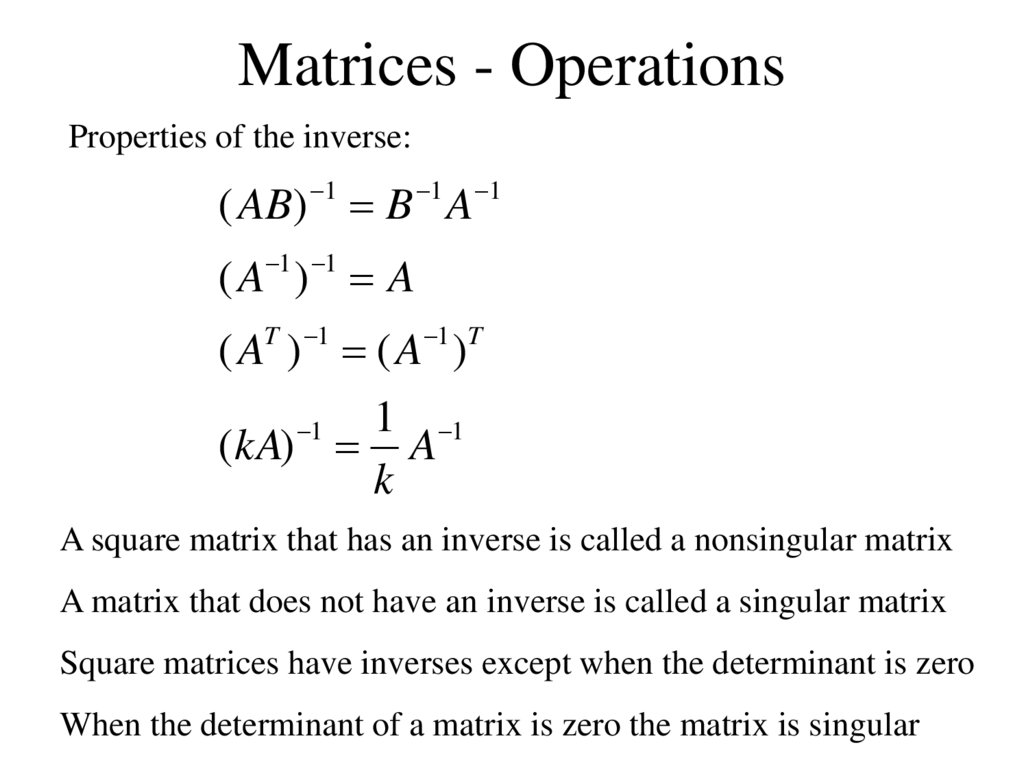
Singular Matrix Properties: For a singular matrix a, row reduction of in does not produce in to the left of the augmentation bar.

Source: cf2.ppt-online.org

Ab = in = ba. Reduce matrix to row echelon form 2. Any matrix b with the above property is called an inverse of a. Are the following matrices singular? For example, if we take a matrix x, whose elements of the first column are zero.

Source: slideplayer.com

Is the conjugate transpose or hermitian transpose of the matrix. Has determinant zero, then this corresponds to the parallelepiped being. Now, the only way this can happen is if, during row reduction, we reach a column whose main.

Source: story-thumbnails.s3.amazonaws.com

For a singular matrix a, row reduction of in does not produce in to the left of the augmentation bar. Home > matrix & vector calculators > singular value decomposition (svd) of a matrix calculator. If you have a set of simultaneous equations

Source: img.yumpu.com

Reduce matrix to row echelon form 2. A bisymmetric matrix is symmetric, persymmetric and centrosymmetric. Now, the only way this can happen is if, during row reduction, we reach a column whose main.

Fonte: Singular Matrix Properties